The energy posses by a body by virtue of its motion is referred to as ‘Kinetic Energy’.
Formula:
K.E. = 1/2 mv2
Kinetic energy depends upon the mass and velocity of body.
If velocity is zero then K.E. of body will also be zero.
Kinetic energy is a scalar quantity like other forms of energies.
Related Posts:
Proof:
Consider a body of mass “m” starts moving from rest. After a time interval “t” its velocity becomes V.
If initial velocity of the body is Vi = 0 ,final velocity Vf = V and the displacement of body is “d”. Then
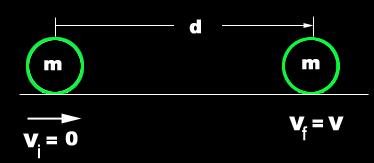
First of all we will find the acceleration of body.
Using equation of motion
2aS = Vf2 – Vi2
Putting the above mentioned values
2ad = V2 – 0
a = V2/2d
Now force is given by
F = ma
Putting the value of acceleration
F = m(V2/2d)
As we know that
Work done = Fd
Putting the value of F
Work done = (mv2/2d)(d)
Work done = mV2/2
OR
Work done = ½ mV2
Since the work done is motion is called “Kinetic Energy”
i.e.
K.E. = Work done
OR
K.E. =1/2mV2
Derivation of Work-Energy Theorem using a Constant Net Force
The energy associated with the work done by the net force does not disappear after the net force is removed (or becomes zero), it is transformed into the Kinetic Energy of the body. We call this the Work-Energy Theorem.
Frame of Reference:
Direction of the Net Force.
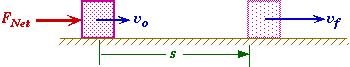
Start with Newton 2nd Law for one-dimensional motion:
Fnet = ma
Next use the Equations for Constant Acceleration that does not involve time:
2as = v2f – v2o => as = v2f – v2o / 2
Calculate the Net Work using the above relationships:
WNet = FNet s=mas = m v2f – v2o / 2
= ½ mv2f – ½ mv2o
= KEf – KEo = ΔKE
Many problems you encounter related to Work and Energy will have constant forces. While you are trying to learn how to use the concepts of Work and Energy, avoid using Newton’s Second Law to solve these problems or else you will have missed the opportunity to learn how to use Work and Energy to solve them.



